The equation of the directrix is x = -4 and the focus coordinates are (1, 3).
Since the directrix is a vertical line, we can use the model below for a parabola that opens to the right or left:
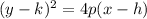
Where the focus is located at (h + p, k) and the directrix is x = h - p.
Since the directrix is x = -4 and the focus is (1, 3), we have:

Adding the first two equations, we have:
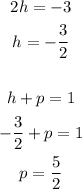
Therefore the equation is:
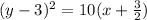
Now, let's rewrite it in the vertex form:
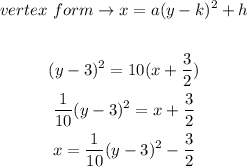
Therefore the correct option is A.