Question

A garden is in the shape of a rectangle and a semicircle, shown below. Determine the area of the garden. Use 3.14 for pi the numbers are . 17 m 12 m

Answer 1

To find the area of the garden find the sum of the area of the rectangle and half the area of the circle.

To find the area of the rectangle use 12 and 17 as the base and the height respectively:

`\begin{gathered} A=b\cdot h \\ A=12\cdot17 \\ A=204 \end{gathered}`

To find the area of the rectangle use 6 as the radius, which is half the diameter, that has a value of 12. Remember this area must be divided by 2 because we only need the area of the semicircle, not the one of the full circle.

`\begin{gathered} A=\pi\cdot r^2 \\ A=3.14\cdot6^2 \\ A=113.04 \\ (A)/(2)=56.52 \end{gathered}`

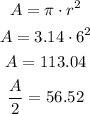
Now, add both the area of the rectangle and the area of the semicircle.

`\begin{gathered} Ag=204+56.52 \\ Ag=260.52 \end{gathered}`

The area of the garden is 260.52 m^2.