Question

Gianna is deciding between two landscaping companies for her place of business. Company A charges $50 per hour and a $150 equipment fee. Company B charges $25 per hour and a $250 equipment fee. Let AA represent the amount Company A would charge for tt hours of landscaping, and let BB represent the amount Company B would charge for tt hours of landscaping. Graph each function and determine the number hours, t,t, that would make the cost of each company the same.

Answer 1

We are given that company A charges $50 per hour and a fixed fee of $150. The total cost "Ca" is then given by the product of the number of hours "t" by the fee per hour plus the fixed fee. This is written mathematically as:

`C_a=50t+150`

Company B charges $25 per hour and a fixed fee of $250. If Cb is the cost for company B, then we have:

`C_b=25t+250`

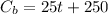
To determine the number of hours "t" for when the cost is the same, then we need to set both costs equal:

`C_a=C_b`

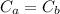
Now we substitute the expression for each cost:

`50t+150=25t+250`

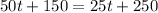
Now we solve for "t", first by subtracting "25t" from both sides:

`\begin{gathered} 50t-25t+150=250 \\ 25t+150=250 \end{gathered}`

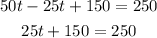
Now we subtract 150 from both sides:

`\begin{gathered} 25t=250-150 \\ 25t=100 \end{gathered}`

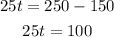
Now we divide both sides by 25:

`t=(100)/(25)=4`

Therefore, the cost for both companies is the same after 4 hours.

To graph the functions we need to have into account that both equations represent lines since they are of the form:

`y=mx+b`

Where "m" is the slope and "b" is the y-intercept. Since the equations are lines we need to determine two points in each one of them.

Let's take the equation for compañy "a" and we replace the value t = 0, we get:

`\begin{gathered} C_a=50(0)+150 \\ C_a=150 \end{gathered}`

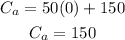
Therefore, the point (0, 150) is in the line for company "a". Now we substitute t = 1, we get:

`\begin{gathered} C_a=50(1)+150 \\ C_a=200 \end{gathered}`

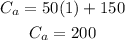
Therefore, the point (1, 200) is also on the line. Now we plot the two points and join them with a line to get the graph. It looks like this:

Now we use the same procedure for company B and we get the following graph:

We notice that the interception point between the lines is the point where the costs are the same.