Question

The equation is: -4.9t^2 + 24.5t + 117.6 = 019. Solve the equation you chose in question 18 to determine when the ball will hit the ground. t = 8 secondst = 4 secondst = 3 secondst = -3 secondsThe ball will never reach the ground.

Answer 1

t = 8 seconds

Explanation:

Given the equation:

`-4.9t^2+24.5t+117.6=0`

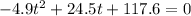
First, multiply all through by 10 in order to change the decimals to whole numbers as it is easier to work with whole numbers.

`\begin{gathered} 10(-4.9t^2+24.5t+117.6)=10*0 \\ -49t^2+245t+1176=0 \end{gathered}`

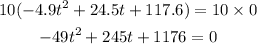
Next, factor out 49.

`\implies49(-t^2+5t+24)=0`

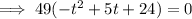
Divide both sides by 49:

`\begin{gathered} (49(-t^2+5t+24))/(49)=(0)/(49) \\ \implies-t^2+5t+24=0 \end{gathered}`

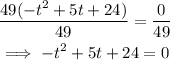
So, we have succeeded in reducing the initial equation given to the form above.

Next, factorize to solve for t.

`\begin{gathered} -t^2+5t+24=0 \\ -t^2+8t-3t+24=0 \\ -t(t-8)-3(t-8)=0 \\ (-t-3)(t-8)=0 \\ \implies-t-3=0\text{ or }t-8=0 \\ \implies t=-3=0\text{ or }t=8 \\ \text{But }t\\eq-3 \\ \implies t=8\text{ seconds} \end{gathered}`

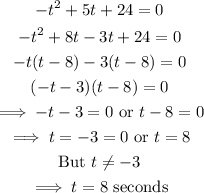
The ball will hit the ground after 8 seconds.