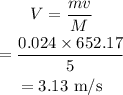The mass of rifle is M = 5kg . It fires the bullet of mass m =24 g = 0.024 kg
It travels a distance, d = 1.5 m and the time taken is t = 0.0023 s
The speed of the bullet will be
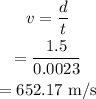
Let the recoil velocity be V. The initial velocity will be zero as rifle and bullet are at rest.
According to the conservation of momentum
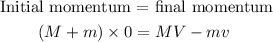
Here, rifle and bullet travel in opposite directions.
Substituting the values, the recoil velocity will be