Question

A fruit company delivers its fruit in two types of boxes: large and small. A delivery of 8 large boxes and 3 small boxes has a total weight of 142 kilograms. A delivery of 2 large boxes and 5 small boxes has a total weight of 61 kilograms. How much does each type of box weigh?

Answer 1

Given,

8 large boxes and 3 small boxes have a total weight of 142 kg

2 large boxes and 5 small boxes have a total weight of 61 kg.

Let us assume that the weight of 1 large box is x.

And the weight of 1 small box is y.

Thus the equation for the given problem can be written as

`\begin{gathered} 8x+3y=142\text{ }\rightarrow\text{ (i)} \\ 2x+5y=61\text{ }\rightarrow\text{ (i}i) \end{gathered}`

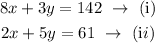
On multiplying the equation (ii) by 4,

`8x+20y=244\text{ }\rightarrow\text{ (i}ii)`

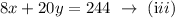
On subtracting equation (i) from equation (ii),

`\begin{gathered} 8x-8x+20y-3y=244-142 \\ \Rightarrow17y=102 \\ \Rightarrow y=6\text{ kg} \end{gathered}`

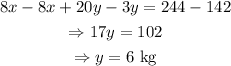
Thus the weight of one small box is 6 kg

On substituting the values of y in equation (ii)

`\begin{gathered} 2x+5*6=61 \\ \Rightarrow2x=61-30 \\ \Rightarrow x=(31)/(2) \\ \Rightarrow x=15.5\text{ kg} \end{gathered}`

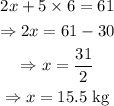
Thus the weight of one large box is 15.5 kg