Answer:
• (4x-1)(3x+6)
,
• 12x²+21x-6
Step-by-step explanation:
From the diagram
Width of the rectangular room = 3x+6
Length of the rectangular room = 4x-1
Area of a rectangle = Lenght x Width
So, an expression that represents the area of the rectangular room is:
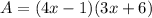
To rewrite the expression as a polynomial in standard form, we expand:
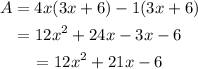
The polynomial in standard form is: 12x²+21x-6