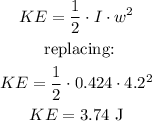ANSWER:
Rod A: 3.74 J
Rod B: 3.74 J
Explanation:
Rod A:
Length of the Rod = l = 0.82 m
Mass of particle attached = m = 0.63 kg
Moment of inertia of the system about given axis:
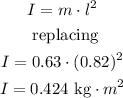
Angular speed of the rod = 4.2 rad/s
Kinetic energy of the rod is:
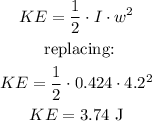
Rod B:
Length of the Rod = l = 0.82 m
Mass of particle attached = m = 0.63 kg
Moment of inertia of the system about given axis:
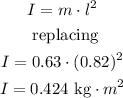
Angular speed of the rod = 4.2 rad/s
Kinetic energy of the rod is: