Question

What is the equation of a quadratic function y = f(x) with two irrational zeros, -√h and √h, where h is a rational number?

Answer 1

We want to write the equation of a quadratic function with two irrational zeros, -√h and √h where h is a rational number.

As the zeros are irrational, we know that what is inside the square roots must be negative. Thus, h is less than zero.

Now we will write the equation, by remembering the factor theorem. We can write the polynomial as:

`f(x)=(x-x_1)(x-x_2)`

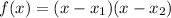
Where x₁ and x₂ are the roots of the function, in this case, -√h and √h. This means that f can be written as:

`\begin{gathered} f(x)=(x-(-\sqrt[]{h}))(x-\sqrt[]{h}) \\ =(x+\sqrt[]{h})(x-\sqrt[]{h}) \\ =x^2-(\sqrt[]{h})^2 \\ =x^2-h \end{gathered}`

This means that the polynomial with the irrational zeros -√h and √h is f(x)=x²-h, where h is a negative rational.

²