Question

Escape speed is the speed required for an object to leave Earth's orbit. It is also the minimum speed anncoming object must have to avoid being captured and pulled into an orbit around Earth. The escape speed forprojectile launched from Earth's surface is 11.2 km/s. Suppose a meteor is pulled toward Earth's surface and,is a meteorite, strikes the ground with a speed equal to this escape speed. If the meteorite has a diameter ofbout 3 m and a mass of (2.3 x 10^5) kg, what is its kinetic energy at the instant it collides with Earth's surface?

Answer 1

Given:

The velocity of meteorite is

`\begin{gathered} v=(11.2km)/(s) \\ =11.2*1000\text{ m/s} \\ =11200\text{ m/s} \end{gathered}`

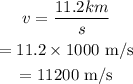
The mass of the meteorite is

`m\text{ = 2.3}*1^{}0^5kg`

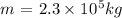
To find the kinetic energy of the meteorite.

Step-by-step explanation:

The kinetic energy is calculated by the formula

`K\mathrm{}E\text{. =}(1)/(2)mv^2`

On substituting the values, the kinetic energy will be

`\begin{gathered} K\mathrm{}E\text{. = }(1)/(2)*2.3*10^5*(11200)^2 \\ =1.44\text{ }*10^(13)\text{ J} \end{gathered}`

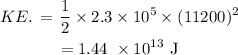
Final Answer: The kinetic energy of the meteorite is 1.44 x 10^(13) J.