Question

Which equation describes the function in the table below a. y = 1.3 + 10

Answer 1

From the table we notice that the change in x is always 5, and the change in y is always 6.5, then this is a line.

The equation of a line is given by:

`y=mx+b`

where m is the slope and b is the y-intercept.

From the table we notice that b=10, this comes from the fact that the y-intercept happens when x=0.

Now, the slope is given by:

`m=(y_2-y_1)/(x_2-x_1)`

using the first two points on the table we have:

`\begin{gathered} m=(16.5-10)/(5-0) \\ =1.3 \end{gathered}`

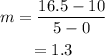
plugging the values into the equation we have:

`y=1.3x+10`

Therefore the answer is A.