Question

Two equally charged, 4.433 g spheres are placed with 2.222 cm between their centers. When released, each begins to accelerate at 240.695 m/s2. What is the magnitude of the charge, in micro-Coulombs, on each sphere?

Answer 1

Given:

The mass of the spheres is m1 = m2 = 4.433 g

The distance between the spheres is d = 2.222 cm

The acceleration is a = 240.695 m/s^2

To find the magnitude of the charge in micro-Coulombs.

Step-by-step explanation:

The magnitude of charge can be calculated by the formula

`F=(kq^2)/(d^2)`

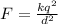
The force can be calculated using Newton's law

`\begin{gathered} F=ma \\ =(m1+m2)a \end{gathered}`

On equating the forces, the charges will be

`\begin{gathered} (m_1+m_2)a=(kq^2)/(d^2) \\ q=\sqrt{((m1+m2)ad^2)/(k)} \\ =\sqrt{((4.433*10^(-3))*240.695*(2.22*10^(-2))^2)/(9*10^9)} \\ =\text{ 2.42}*10^(-7)\text{ C} \\ =0.242\text{ }\mu\text{ C} \end{gathered}`

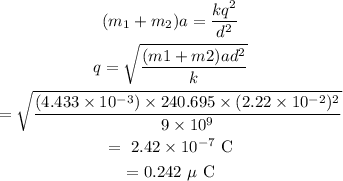
Thus, the magnitude of the charge is 0.242 micro Coulomb.