Given data:
The given coordinates of the pentagon are M(-4,1),N(-2,3). O(0, 3), P(4, 3), and Q(2,-7).
The coordinatte after 90 degrees counterclockwise rotation is,
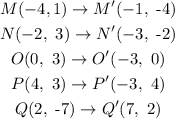
The final coordinates after 2/3 dilation factor is,
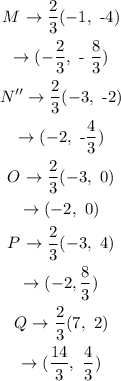
Thus, the final coordinates after transformation are M''(-2/3, -8/3), N''(-2, -4/3). O''(-2, 0), P''(-2, 8/3), and Q''(14/3, 4/3).