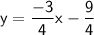Question

What is the equation of the line that is parallel to thegiven line and has an x-intercept of -3?

Answer 1

`\sf y = (-3)/(4)x-(9)/(4)`

Explanation:

Equation of line: y = mx + b

Here m is the slope and b is the y-intercept.

First, let us find the slope of given line.

(-4 ,4) & (4 , -2)

`\sf \boxed{Slope =(y_2-y_1)/(x_2-x_1)}`

`\sf = (-2-4)/(4-[-4])\\\\\\=(-6)/(4+4)\\\\\\=(-6)/(8)\\\\\\=(-3)/(4)`

Parallel lines have same slope.

m = -3/4

Equation of the line:

`\sf y =(-3)/(4)x+b`

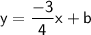
At x_intercept, y is 0. (-3 , 0). The line passes through the point (-3 ,0).

Substitute in the above equation and we can find the value of 'b'.

`\sf 0 = (-3)/(4)*(-3)+b\\\\\\0 = (9)/(4)+b\\\\\\b = (-9)/(4)`

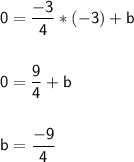
Equation of the required line:

`\sf y =(-3)/(4)x-(9)/(4)`