We have two equations, since both of them are linear in x and y we conclude that this is are lines. To find their slope we need to write them in the slope-intercept form:

Line x-y=2
Let's write the equation in slope intercept form:
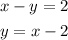
Comparing the last line with the slope intercept form we conclude that the slope of this line is 1.
Now, to find the values of y given a value of x we just need to plug the value of x we want and find its corresponding value of y with the equation; for example, if x=0, then we have:
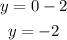
Following this procedure we have that:
To graph the line we plot this points on the plane and join them with a straight line:
Line 3x-4y=9
Following the same procedure as with the previous line we have that this line can be written as:
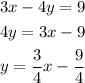
Hence the slope of this line is 3/4.
The table we get in this case is:
Plotting this points and joining with a straight line we get the graph of the equation:
Graphical solution.
To find the solution of the system graphically we need to graph both lines in the same plane; the solution of the system is the point where the lines intersect. Let's do that:
From the graph we notice that the intersection happens at the point (-1,-3).
Therefore, the solution of the system is the point (-1,-3) which means that x=-1 and y=-3
Algebraic solution.
To find the solution of the system:
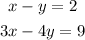
we solve the first equation for y:

and plug this in the second equation:
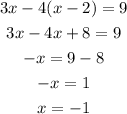
once we have the value of x we plug it in the expression of y:
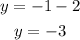
Therefore, we have that x=-1 and y=-3 just like we gound graphically