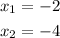The Vertex form of a Quadratic function is:
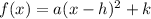
Where the vertex of the paraboola is:
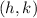
Given the following Quadratic equation:

You need to convert it into Vertex form by completing the square. The steps are shown below:
- Identify the coefficient of the term with the variable "x". In this case, this is:

- Divide it by 2 and square it:
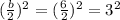
- Add and subtract the number obtained (in the right side of the equation):
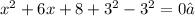
- Order it and express it as a square of a binomial, you get:
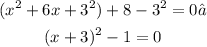
- Add 1 to both sides of the equation:
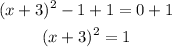
To solve the equation you must take the square root of both sides of the equation:
![\begin{gathered} \sqrt[]{(x+3)^2}=\pm\sqrt[]{1} \\ \\ x+3=\pm1 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/sg5647f2groq85969p3hhruv5lbzpiq5d9.png)
Finally, subtract 3 from both sides of the equation:
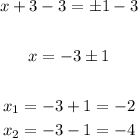
The answer is:
- Vertex form:

- Solutions: