A piece of wire is cut into two pieces. That means each part would be assigned an unknown variable. Let one part be x and the other part be y.
That means,
![x+y=15\operatorname{cm}]()
If the longer part is x, and the longer part is 3cm longer than the shorter part, then we would have the following;
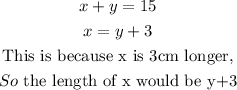
We can now refine the equation as follow;
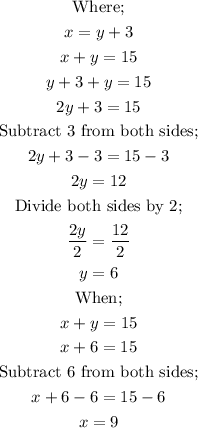
ANSWER:
The length of the shorter piece of wire is 6cm