Question

Given that ax²+bx+c= 0 and that a, b, and c are integers, match each of the following values of the discriminant to the type of solutions. The discriminant is -3 two real rational N [ Select] The discriminant is 9 one real two imaginary The discriminant is 3 two real rational two real irrational The discriminant is O

Answer 1

The expression for Discriminant is :

`Discri\min ant=b^2-4ac`

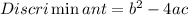
The expression of roots with discriminant is :

`x=\frac{-b\pm\sqrt[]{D}}{2a}`

1) Discrminant is ( - 3)

Then The root will be negative :

`\begin{gathered} x=\frac{-b\pm\sqrt[]{D}}{2a} \\ x=\frac{-b\pm\sqrt[]{(-3)}}{2a} \end{gathered}`

Thus there are no real roots

Discriminant is ( - 3 ) , Thre are no real roots, both the roots are imaginary

Two Imaginary roots

2) Discriminant is 9

Then the roots will be :

`\begin{gathered} x=\frac{-b\pm\sqrt[]{D}}{2a} \\ x=\frac{-b\pm\sqrt[]{(9)}}{2a} \end{gathered}`

There will be two real roots

Discriminant is 9, There are two real roots

3) Discriminant is 3 :

Then the roots will be :

`\begin{gathered} x=\frac{-b\pm\sqrt[]{D}}{2a} \\ x=\frac{-b\pm\sqrt[]{(3)}}{2a} \\ as\text{ :}\sqrt[]{3}\text{ is a irrational number } \\ x\text{ = irrat}ionals\text{ number} \end{gathered}`

There will be two real irrational roots

4) Discriminant is zero

`\begin{gathered} x=\frac{-b\pm\sqrt[]{D}}{2a} \\ x=\frac{-b\pm\sqrt[]{(3)}}{2a} \\ as\text{ :}\sqrt[]{3}\text{ is a irrational number } \\ x\text{ = irrat}ionals\text{ number} \end{gathered}`