Answer:
23.6
Explanation:
Hello There!
So they want us to find ∠Z
We are given ∠Zs opposite side length and the length of the hypotenuse
Sine refers to opposite divided by hypotenuse
So we can use sine to solve for ∠Z
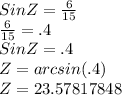
Note: arcsin is the inverse of sin
Other way we could find ∠Z:
We could also use law of sines which states that a side length divided by sin(its opposite angle) is equal to a different side length divided by sin ( its opposite angle)
So specifically for this triangle

step 1 multiply each side by 6
note : flip the fraction
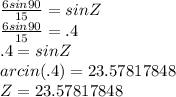
Then our final step is to give our answer to the first decimal place
so the answer would be 23.6