We know that function g is defined as:

where
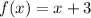
This means that the factors of the function g(x) are x and x+3; now, for the intervale (-8,-3) we notice that this factors are always negative and then their product would be positve; therefore we conclude that function g(x) is positive for the interval (-8,-3) because its two factors are negative in that interval.
Now, the function g(x) is decreasing in this interval because we expect the factors to change sign in another interval, this can be seen in the graph, eventually the function would be negative and then again it would be positive; this means that function necessarily changes its behavior in some intervals. To proof this we can use the derivative of the function g(x):
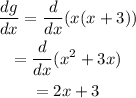
We notice that the derivative of the function g(x) is negative for the interval (-8,-3); this means that in this interval the function is decreasing.