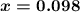Question

Solve the following exponential equation. Express irrational solutions in exact form and as a decimal rounded to three decimal places.What is the exact answer? Select the correct choice below and, if necessary, fill in the answer box to complete your choice.(Simplify your answer. Type an exact answer.)What is the answer rounded to three decimal places? Select the correct choice below and, if necessary, fill in the answer box to complete your choice.(Simplify your answer. Type an integer or decimal rounded to three decimal places as needed.)

Answer 1

To solve the exponential equation, we apply natural logarithm from both sides of it:

`\begin{gathered} 4^(1-9x)=5^x \\ \ln (4^(1-9x))=\ln (5^x) \end{gathered}`

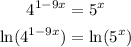
Now, we apply the power rule of the logarithms:

`\log _b(M^p)=p\cdot\log _b(M)\Rightarrow\text{ Power rule}`
`\begin{gathered} \ln (4^(1-9x))=\ln (5^x) \\ (1-9x)\ln (4^{})=x\ln (5^{}) \end{gathered}`

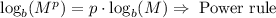
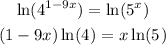
Now, we apply the distributive property on the left side of the equation:

`\begin{gathered} 1\cdot\ln (4)-9x\cdot\ln (4^{})=x\ln (5^{}) \\ \ln (4)-9x\ln (4^{})=x\ln (5^{}) \end{gathered}`

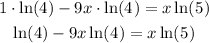
Now, we subtract xln(5) from both sides of the equation:

`\begin{gathered} \ln (4)-9x\ln (4^{})-x\ln (5)=x\ln (5^{})-x\ln (5) \\ \ln (4)-9x\ln (4^{})-x\ln (5)=0 \end{gathered}`

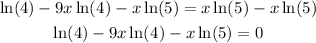
Now, we subtract ln(4) from both sides:

`\begin{gathered} \ln (4)-9x\ln (4^{})-x\ln (5)-\ln (4)=0-\ln (4) \\ -9x\ln (4^{})-x\ln (5)=-\ln (4) \end{gathered}`

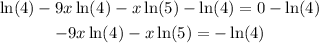
Now, we factor x on the left side:

`\begin{gathered} x(-9\ln (4^{})-\ln (5))=-\ln (4) \\ \text{ Divide by }-9\ln (4)^{}-\ln (5)\text{ from both sides} \\ \frac{x(-9\ln(4^{})-\ln(5))}{(-9\ln(4^{})-\ln(5))}=\frac{-\ln(4)}{(-9\ln(4^{})-\ln(5))} \\ x=\frac{-\ln(4)}{(-9\ln(4^{})-\ln(5))} \\ x=\frac{-\ln(4)}{-(9\ln(4^{})+\ln(5))} \\ x=\frac{\ln(4)}{9\ln(4^{})+\ln(5)} \end{gathered}`

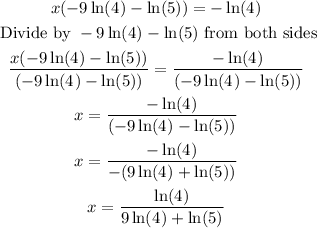
Thus, the exact solution is:

`$$\boldsymbol{x=\frac{\ln(4)}{9\ln(4^{})+\ln(5)}}$$`

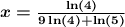
And the solution rounded to three decimal places is:

`$$\boldsymbol{x=0.098}$$`