Triangle LRG is similar to triangle CNP. Therefore, the following ratios apply;
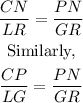
Hence, for triangle LRG to be dilated to become CNP,

Therefore, the scale factor needed to dilate triangle LRG so that its image is congruent to triangle CNP is 1.5 (that is the decimal equivalent of 3/2)
This means triangle LRG would be multiplied by 1.5 in order to have an image congruent to triangle CNP