The general form of the equation of a line is given by;

where m is the slope of the line and b is the y-intercept.
You have the following equation of a line:
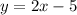
a) By comparing the previous equation with the general form of the equation of a line, you can notice that the slope of the line is m = 2.
b) Any equation of a line with the same slope will be parallel to the line
y = 2x - 5.
c) You use the following formula for the slope of a line:
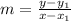
where (x1,y1) is a point of the line.
If you replace the given point (-2 , -1) in the previous equation, if you also replace the value of m and solve for y, you obtain:
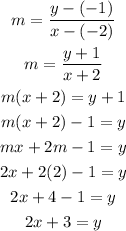
Which is the same as:
y = 2x + 3
Hence, the equation y = 2x + 3 is a line parallel to the line y = 2x - 5 and passes trough the point (-2,-1)