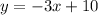Recall that the slope intercept equation of a line is of the form y=mx+b where m is the slope and b is the y intercept. In general, two lines are parallel if they look like this
This translates to the fact the the lines never touch each other. This also means that, in a way, the lines increase (or decrease) at the same rate. This notion of rate of increase/decrease is captured by the fact that if we have lines y=m1x+b1 and y=m2x+b2 they are parallel if they have the same slope. That is
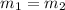
We are given the line y=-3x+4 (with slope -3 and y intercept 4) and we want to find the equation y=mx+b. Since we want that both lines are parallel, based on our previous analysis, we should have
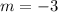
So, so far, our equation looks like this
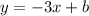
Now, we want this line to pass through the point (1,7), this means that whenever x=1, we must have y=7. So, we have the following equation
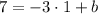
If we add 3 on both sides, we get
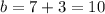
So our final result is the equation