1. We are given that the angle is determined by the formula
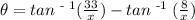
lets say we want to sit at x = 25 feet. So if we replace x with 25 and we plug it in our equation, using a calculator in radians we get

3. To transform one angle that is in radians, to degrees, we must mutiply by 180° and divide by pi radians. So, in our case we get
