A principal $7000, rate 5%=0.05, and time 2 years is given.
It is stated that it is compounded semiannually, that is, twice in a year.
The question requires that you calculate the amount in the account after 2 years and the interest earned.
The formula for the amount (compound interest) is given as:

Where,
• A is the final amount.
,
• P is the principal
,
• r is the rate
,
• n is the number of times interest is compounded annually.
,
• t is the time in years.
In this case P=7000, r=0.05, n=2, t=2. Substitute these values into the formula:
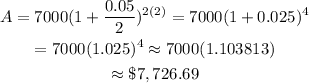
The equation that relates the amount, A, principal, P, and interest earned, I is given as:
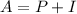
Substitute A=7,726.69, P=7000 into the formula:
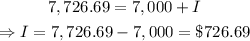
It follows that:
The amount of money in the account after 2 years is about $7,726.69.
The amount of interest earned is about $726.69.