Question

The marching band is learning a new formation. There are 22people in the first row, 33 people in the second row, 44 people inthe third row, and so on. How may people will be in the 7th row?If there are only 7 rows, how many people are in the band?

Answer 1

We can see that the number of people in each row has a linear relationship with the row number.

In the row 1 the number of people is 22, in the row 2 there are 33 people, 11 more than row 1. In the row 3, there are 44 people, 11 more than row 2.

So, the function is:

`\begin{gathered} \text{people}=22+(n-1)\cdot11 \\ \text{people}=2\cdot11+(n-1)\cdot11 \\ \text{people}=11\cdot(n-1+2) \\ \text{people}=11\cdot(n+1) \\ \text{Where n is the number of row} \end{gathered}`

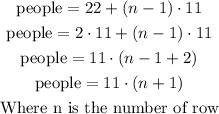
We can use the equation above to find the number of people in row 7:

`\text{people in row 7}=11\cdot(7+1)=11\cdot8=88`

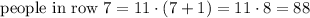
The number of people from row 1 to 7 is:

`\begin{gathered} \text{Total}=\sum ^7_(n\mathop=1)11\cdot(n+1)=11\cdot(\sum ^7_(n\mathop=1)n+\sum ^7_(n\mathop=1)1)=11\cdot((7\cdot(7+1))/(2)+7) \\ \text{Total}=11\cdot(7\cdot(8)/(2)+7) \\ \text{Total}=11\cdot(7\cdot4+7) \\ \text{Total}=11\cdot35=385 \end{gathered}`

The total number of people is 385