Question

The graph of the following function has two slant asymptotes.

Answer 1

1) We can find the slant asymptotes by working with limits. So let's begin with that, by calculating the side limits of this function to check its behavior.

`\begin{gathered} \lim _(x\to-\infty\: )(f\left(x\right))/(x)\: \Rightarrow\lim _(x\to-\infty\: )(√(25+36x^2))/(x)=(√(25+36x^2))/(x) \\ \lim _(x\to\: -\infty\: )\mleft(-\sqrt{(25)/(x^2)+36}\mright) \\ -\sqrt{\lim_(x\to\:-\infty\:)\left((25)/(x^2)+36\right)} \\ -\sqrt{\lim_(x\to\:-\infty\:)\left((25)/(x^2)\right)+\lim_(x\to\:-\infty\:)\left(36\right)} \\ \lim _(x\to\: -\infty\: )\mleft((25)/(x^2)\mright)=0 \\ \lim _(x\to\: -\infty\: )\mleft(36\mright)=36 \\ \mathrm{Simplify\: }-√(0+36)=-6 \\ y=-6x \\ y=6x\mathrm{\: }\mleft(\mathrm{slant}\mright),\: y=-6x\mathrm{\: }\mleft(\mathrm{slant}\mright) \end{gathered}`

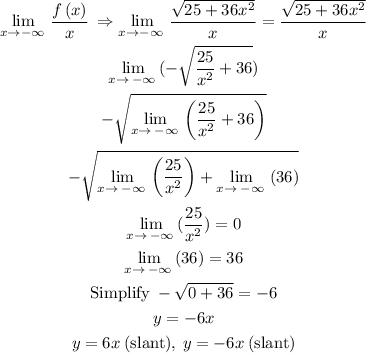
Note that here we performed some limits properties, a bit simplified due to the time. But the point here is the behavior of this function.

2) As we know the slant asymptotes are y=6x and y=-6x so we cn