1 Answer

Ask a Question

Darcyy · Answer 1 · 2023-02-26T12:10:03+0000

Since two times a number decreased by four is between -14 and 32, we need to solve the following compound inequality:

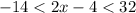
In order to solve it, we can apply the same operations on all the parts of the inequality, until we isolate the variable x and find its value.

We obtain:

$\begin{gathered} -14+4<2x-4+4<32+4 \\ \\ -10<2x<36 \\ \\ -(10)/(2)<(2x)/(2)<(36)/(2) \\ \\ -5Now, we need to write the answer using interval notation. Since x can't be -5 nor 18, only the values between them, the interval of valid values is:[tex](-5,18)$

0 Comments

Please log in or register to add a comment.

Please log in or register to answer this question.

1 Answer

0 Comments

Please log in or register to add a comment.

Other Questions