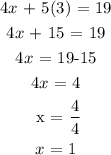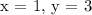
Here, we want to solve the system of linear equations simultaneously
We start by multiplying the first equation by 2 and the second by 1
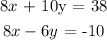
We can now proceed to subtract the second equation from the first
That will give;
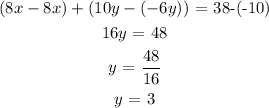
To get the value of x, we will need to substitute the calculated value of y into any of the two initial equations
Thus, we have it that;