Question

The doubling time of an investment earning 4% interest if interest is compounded continuously is ? Years

Answer 1

17.3 years

Explanation:

For an investment compounded continuously, the amount, A(t) in the account after a period of t is given by:

`A(t)=A_oe^(rt)`

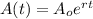
• When the initial amount, Ao is doubled, A(t)=2Ao

,

• Interest Rate = 4% =0.04

Substitute these values into the equation:

`2A_0=A_oe^(0.04t)`

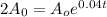
We solve the equation for t:

`\begin{gathered} \text{Divide both sides by }A_0 \\ (2A_0)/(A_o)=(A_oe^(0.04t))/(A_o) \\ e^(0.04t)=2 \\ \text{Take the natural log \lparen ln\rparen of both sides} \\ ln(e^(0.04t))=\ln(2) \\ 0.04t=\ln(2) \\ \text{ Divide both sides by 0.04} \\ t=(\ln(2))/(0.04) \\ t=17.3\text{ years} \end{gathered}`

The doubling time is 17.3 years (rounded to the nearest tenth).