The Solution:
Given the data below:
28, 27, 26, 25, 32, 26, 30, 25, 30, 28, 28, 30.
Required:
To find the range and the interquartile range.
Step 1:
Arrange the given data in ascending order.
25, 25, 26, 26, 27, 28, 28, 28, 30, 30, 30, 32
The range is the difference between the smallest number and the largest number.
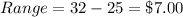
Step 2:
Find the interquartile range.
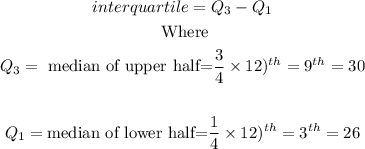
So,
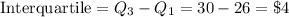
Therefore, the correct answers are:
Range = $7.00
Interquartile range = $4.00