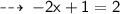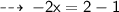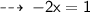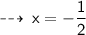Question

Helppp! Solve for x!​

Answer 1

`\qquad \qquad\huge \underline{\boxed{\sf Answer}}`

Here we go ~

`\qquad \sf  \dashrightarrow \: 6 {}^( - 2x + 1) = 36`

`\qquad \sf  \dashrightarrow \: {6}^( - 2x + 1) = {6}^(2)`

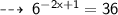
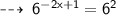
now, let's apply logarithm on both sides with base (6)

`\qquad \sf  \dashrightarrow \: log_(6)( 6 {}^( - 2x + 1) ) = log_(6)( {6}^(2) )`

According to properties of logarithm, the exponent on the number come out and it's written as a product of exponent times the logarithm.

that is :

`\sf [ log_(a)(b {}^(n) ) = n * log_( a )(b) ]`

`\qquad \sf  \dashrightarrow \: ( - 2x + 1) * log_(6)(6) = 2 * log_(6)(6)`

now, as we know : when the base and argument of log are same, then value of log is 1

`\qquad \sf  \dashrightarrow \: - 2x + 1 = 2`

`\qquad \sf  \dashrightarrow \: - 2x = 2 - 1`

`\qquad \sf  \dashrightarrow \: - 2x = 1`

`\qquad \sf  \dashrightarrow \: x = - (1)/(2)`

Answer 2

• 
  `6^(-2x + 1) = 36`
• =>
  `(6)6^(-2x) = 36`
• =>
  `6^(-2x) = 6`
• =>
  `-2x = 1`
• =>
  `x = (-1)/(2)`

The solution for this equation is -1/2.