Question

In this diagram, circle A has radius = 5 and DC = 7.4 BC is tangent line, calculate the distance of BC.

Answer 1

Since the line BC is tangent to the circle we know that the angle CBA is a right angle, hence the triangle is a right one and we can apply the pythagorean theorem.

Now, we know that:

`AB=5`

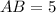
since the radius is 5. Furthermore we also know that:

`\begin{gathered} AD+DC=AC \\ 5+7.4=AC \\ AC=12.4 \end{gathered}`

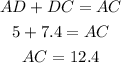
Now, in thie case the pythagoean therorem is:

`AB^2+BC^2=AC^2`

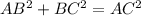
Plugging the values we know and solving for BC we have:

`\begin{gathered} 5^2+BC^2=12.4^2 \\ BC^2=12.4^2-5^2 \\ BC=\sqrt[]{12.4^2-5^2} \\ BC=11.35 \end{gathered}`

Therefore BC is 11.35.