Question

An adult who visited a therapist during the past year is randomly selected. what is the probability this adult used non-prescription antaepressants?Round your answer to 2 decimal places.(b what is the probability that an adult visited a therapist during the past year, given that he or she used non-prescription antidepressants? Round your answer to 2 decimal places.

Answer 1

We will answer the question a) using the following formula:

`P(N|T)=(P(N\cap T))/(P(T))`

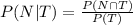
where we have the following event:

`\begin{gathered} N=\text{ Adult use non-prescription antidepressants} \\ T=Adult\text{ visit a therapist} \end{gathered}`

Therefore, the probability P(N|T) is the probability of a randomly selected adult use non-prescription antidepressants given that he visited the therapist. This formula is known as conditional probability formula.

To use the formula, we have to calculate the probability :

`P(N\text{ }\cap T)`

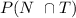
This is the probability of the intersection between the events N and T, that is, the probability that a given adult visits a therapist and use non-prescription antidepressants. Thsi information was given in the question, so we have

`P(N\cap T)=21\%=0.21`

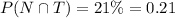
Therefore, we can calculate the probability required in the part a) as :

`P(N|T)=(P(N\cap T))/(P(T))=\frac{21\%\text{ }}{26\%}=(0.21)/(0.26)\approx0.81=81\%\text{ }`

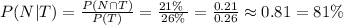
Therefore, the answer for the part a) is 81%, or in decimal number 0.81.

Part b)

We are asked to calculate the probability of that a randomly selected patient who use non-prescription antidepressants visit the therapist. This can be written in symbols as (we use the notations from the solution of the part a))

`P(T|N)=(P(T\cap N))/(P(N))=(21\%)/(43\%)=(0.21)/(0.43)\approx0.49=49\%\text{ }`

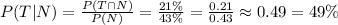
Therefore, the answer for the part b) is 49%, or in decimal number 0.49.