Question

9) An observer standing on the top of a vertical cliff spots a house in the adjacent valley at an angle of depression of 17 degrees. The cliff is 65 m tall. How far is the house from the base of the cliff, to the nearest meter?

Answer 1

213 meters.

Step-by-step explanation:

Height of the cliff = 65 m

The angle of depression = 17 degrees.

A diagram representing this problem is attached below:

We solve for x in the diagram.

`\begin{gathered} \tan \theta=\frac{Opposite}{\text{Adjacent}} \\ \tan 17^0=(65)/(x) \\ x\tan 17^0=65 \\ x=(65)/(\tan 17^0) \\ x=212.6m \\ x\approx213m \end{gathered}`

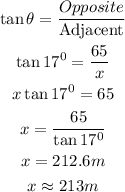
The distance of the house from the base of the cliff, to the nearest meter, is 213 meters.