Let's generate an equation from the relationships found in the given scenario,
We get,
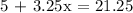
This equation reflects Trina's expenses during her indoor rock climbing. She spent an entrance fee of $5 and a rate of $3.25 per hour climb with a total expense of $21.25.
Where,
x = the number of hours Trina climbed.
Let's now compute how long did Trina use the indoor rock climbing facility.
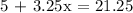
Applying the Rule of Additive Inverse Property, we get,
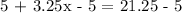
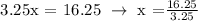
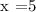
Therefore, Trina spent 5 hours in the indoor rock climbing facility.
If Ralph wants to spend the same amount of money, he must use the indoor rock climbing facility for 5 hours.