Question

What is the five number summary for the data set? 1, 4, 6, 7, 8, 10, 12, 13, 14, 16,19, 22, 23, 27, 30, 31, 31, 33, 34, 36, 41, 42, 47

Answer 1

Step-by-step explanation:

Given the data set:

`1,4,6,7,8,10,12,13,14,16,19,22,23,27,30,31,31,33,34,36,41,42,47`

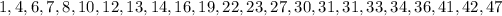
The data set contains 23 items where:

• The minimum number = 1

,

• The maximum number = 47

Next, the median number (the number in the middle will be the 12th item):

`1,4,6,7,8,10,12,13,14,16,19,\boxed{22},23,27,30,31,31,33,34,36,41,42,47`

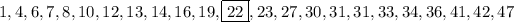
• The median = 22

The lower quartile will be the number in the middle of the first half.

`1,4,6,7,8,\boxed{\textcolor{red}{10}},12,13,14,16,19,\boxed{22},23,27,30,31,31,33,34,36,41,42,47`

• The lower quartile, Q1 = 10

Similarly, the upper quartile will be the number in the middle of the second half.

`1,4,6,7,8,\boxed{\textcolor{red}{10}},12,13,14,16,19,\boxed{22},23,27,30,31,31,\boxed{\textcolor{red}{33}},34,36,41,42,47`

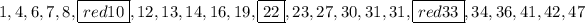
• The upper quartile, Q3= 33

Therefore, the five-number summary is: 1,10,22,33,47 where

• Minimum: 1

,

• Lower Quartile = 10

,

• Median = 22

,

• Upper Quartile = 33

,

• Maximum = 47