By solving the wave equation for a string, we can find out that the velocity of a wave on it is:
![v=\sqrt[\placeholder{⬚}]{(T)/((m)/(L))}](https://img.qammunity.org/2023/formulas/physics/college/4v2rae25evlxrzsysjxcpltq50iubqre9p.png)
Where T is the tension, m is the mass and L is the length. We can calculate in our case, which leaves us with:
![v=\sqrt[\placeholder{⬚}]{(2000)/((100)/(20))}=20(m)/(s)](https://img.qammunity.org/2023/formulas/physics/college/4uc1zddxt5w5nvxvr0jckdegjgpoyzras7.png)
So the velocity in which a wave propagates on this cable is 20 m/s. We can also calculate its fundamental vibration frequency, which is:
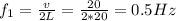
However, this is when there is a single "half-wave" on the cable. As we know from the exercise, the wavelength (i.e. the full wave) is 0.5m, so our cable can fit 20/0.5 or 40 full waves, which is 80 half waves.
With this in mind, we can use the formulas for a standing wave:
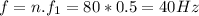
Then, our final answer is f=40Hz