Let the function be,
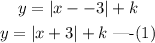
Here, k is the missing value to be found out.
Input missing values
Now, consider the coordinates of a point on the graph.
(x,y)=(-3, -4) is a point on the graph. Hence, substitute x=-3 and y=-4 in equation (1).
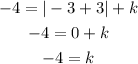
Hence, k=-4.
So, the missing value in the function is -4.
Option B is correct.
Simplified function of graph
Now, put k=-4 in equation (1) to obtain the simplified function of the graph.

Therefore, the simplified function of the graph is y=|x+3|-4.
Hence, option A gives the simplified function of the graph .