Question

Edgar has a birdbath that holds 3 quarts of water. He only wants to fill it 3/4 full. How many 1 pint containers does it take to fill the birdbath? How could he fill it with pint and cup containers? Explain.

Answer 1

a. 5 1-pint containers

b. 4 1-pint containers and 1 1-cup containers

Explanation:

Given:

1 quarts = 2 pints

1 quarts = 4 cups

We know that full equals 3 fourths, so 3/4 of this would be:

`3\cdot(3)/(4)=(9)/(4)\text{ quarts of water}`

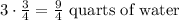
We convert to pints and get the following:

`(9)/(4)\text{quarts}\cdot\frac{2\text{ pints}}{1\text{ quart}}=(9)/(2)\text{ pints of water}=4.5\text{pints of water}`

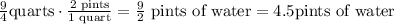
Since we do not have a full 1-pint container, a total of 5 1-pint containers would be needed.

Now, if we want to express the answer in containers of pints and cups we can say that we have 4 containers of 1 pint and the remaining 0.25 of quarts we can express in cups, just like this:

`(1)/(4)\text{quarts of water}\cdot\frac{4\text{ cups}}{1\text{ quart}}=1\text{ cup of water}`

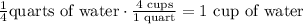
Which means that we need 4 containers of 1 pint and 1 container of a cup