Step-by-step explanation
We know that M is the midpoint of segment EF which means that the two segments created by it, EM and MF have the same length. Then we can use this information to construct an equation for x equalizing the expressions for the lengths of these segments:

Now let's solve this equation for x. We can start by adding 6 to both sides:
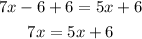
Then we substract 5x from both sides:

And we divide both sides by 2:
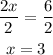
Now that we have x we can find the length of EM, MF and EF:

Answers
Then the answers are:
x = 3
EM = 15
MF = 15
EF = 30