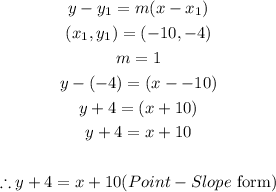Question

Find the equation of the line shown. Enter your answer in point-slope form.434210x510S10510AnswerKeypadKeyboard Shortcuts

Answer 1

We will firstly pick out 2 ordered pairs that lie along the straight line, we have:

`\begin{gathered} (x_1,y_1)=(-10,-4) \\ (x_2,y_2)=(4,10) \end{gathered}`

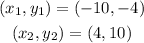
We will proceed to calculate for the slope, we have:

`\begin{gathered} slope,m=(\Delta y)/(\Delta x)=(y_2-y_1)/(x_2-x_1) \\ slope,m=(y_2-y_1)/(x_2-x_1) \\ \text{Substitute the values for these variables into the formula, we have:} \\ slope,m=(10-(-4))/(4-(-10)) \\ slope,m=(10+4)/(4+10) \\ slope,m=(14)/(14) \\ slope,m=1 \end{gathered}`

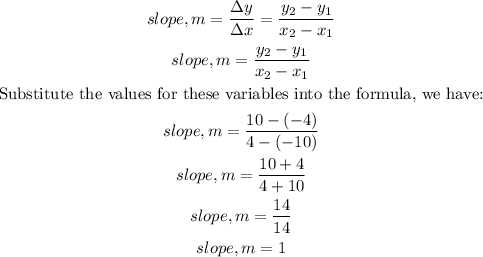
The equation for the general formula of a straight line becomes:

`\begin{gathered} y=mx+b \\ m=1 \\ y=x+b \end{gathered}`

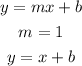
We will proceed using the Point-Slope equation to obtain the equation as shown below:

`\begin{gathered} y-y_1=m\mleft(x-x_1\mright) \\ (x_1,y_1)=(-10,-4) \\ m=1 \\ y-\mleft(-4\mright)=(x--10) \\ y+4=(x+10) \\ y+4=x+10 \\ \\ \therefore y+4=x+10(Point-Slope\text{ form}) \end{gathered}`