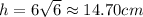Answer
• Hypotenuse: 14.70cm
,
• Height: 12cm
,
• Base: 8.48cm
Step-by-step explanation
As we have the angles of the new triangle formed when the hypotenuse is divided, we can find the base of the original triangle using trigonometric functions, which in our case it would represent the hypotenuse h of the new triangle:
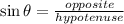
Replacing the values:
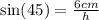
![h=\frac{6cm}{\sin(45\operatorname{\degree})}=6√(8)\approx8.48cm]()
Now we have the base of the original triangle, and we know that the height is 12cm. Then, the hypotenuse is x + 6.
Using the Pythagorean Theorem:

where each parameter is equal to:
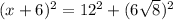
Simplifying:
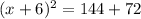
Applying the square root to both sides of the equation:
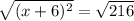

Finally, as:
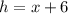
Then: