We have to find the measure of side IJ.
As we now the figures are similar, we know that the proportions between corresponding sides are equal.
We know that the largest sides of each figures (KL and HG) are corresponding. Then, the opposites sides to each one, EF and IJ, are also corresponding sides.
We can then write the proportions as:
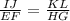
We can replace the values for all the segments and calculate the length of IJ as:
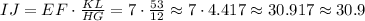
Answer: the length of IJ is 30.9 units.