Question

Please help me with this math problem, The seventh-grade winner ran the mile in 5 minutes and 8 seconds. The eighth-grade winner ran the mile in 4 minutes and 55 seconds. How many more seconds did it take for the seventh-grade winner to run a mile?

Answer 1

First, let’s simply convert minutes to seconds.
5 minutes = 300 seconds
4 minutes =240 seconds

Second, simply add the remaining seconds to each students mile time
7th grader: 308 seconds
8th grader: 295 seconds

Third, simply subtract the smallest amount of time from the largest

308-295=13

Our answer here is 13 seconds.

Answer 2

We will convert minutes to seconds for both times. To do that we will use the following conversion factor:

`1\min =60s`

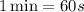
Converting the time for the seventh grade:

`5\min *(60s)/(1\min)=300s`

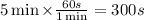
Therefore, the total number of seconds for the seventh grade is:

`t_7=300s+8s=308s`

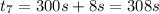
Now we convert the time for the eighth grade:

`4\min *(60s)/(1\min)=240s`

The total number of seconds for the eighth grade is:

`t_8=240s+55s=295s`

Now we determine the difference between both times:

`\begin{gathered} d=t_7-t_8 \\ d=308s-295s=13s \end{gathered}`

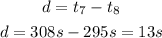
Therefore, the seventh-grade winner has 13 more seconds.

Another way of solving it is noticing that for 4 min and 55s there is a difference of 5 seconds to be 5 min and to that, we add the 8 seconds of the seventh grade we get:

`5s+8s=13s`

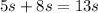
And we get the same answer.