Question

Calculate the minor arc length AB and the major arc length ACB for circle O shown. What do youknow about their sum? Hint: Think circumference.6 cm135C СO 57 cmO 10 cm25 cmO 20 cm

Answer 1

The formula to find the arc length if the angle has been measured in degrees is

`\text{ arc length }=\frac{\theta}{360\text{\degree}}\cdot2\pi r`

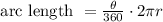
So, for the arc AB, you have

Now, for the arc ACB, you have

Finally, if you add the arc lengths and leave this sum in terms of π, you have

`\begin{gathered} \text{ arc length AB + arc length ACB }=(15)/(4)\pi cm+(25)/(4)\cdot\pi cm \\ \text{Circumference }=((15)/(4)+(25)/(4))\pi cm \\ \text{Circumference }=((15+25)/(4))\pi cm \\ \text{Circumference }=(40)/(4)\pi cm \\ \text{Circumference }=10\pi cm \end{gathered}`

Therefore, if you add the length of the minor arc AB and the length of the major arc ACB, you get the circumference of the circle, whose measure is

`10\pi cm`