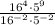5^5(16^2*5^3)^3=?Rewrite the expression as a product of two powers and two quotients

Question

asked Nov 28, 2023 145k views

1 Answer

Dipu · Answer 1 · 2023-12-03T20:30:45+0000

We have the next expression:

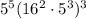
$5^5(16^2\cdot5^3)^3$

Now, solve the parentheses using the next property:

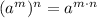
$(a^m)^n=a^(m\cdot n)$

Therefore:

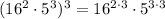
$(16^2\cdot5^3)^3=16^(2\cdot3)\cdot5^(3\cdot3)$
$=16^6\cdot5^9$

Which represents the product of two powers.

But, we need to find two quotients:

Then, we can rewrite the next expression:

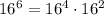
$16^6=16^4\cdot16^2$

To make fractions, we use the next property:

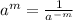
Then:

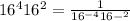
The result expression for the product of two powers:

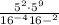
$(5^2\cdot5^9)/(16^(-4)16-^2)$

The result for the product of two quotients:

$(16^4\cdot5^9)/(16^(-2)\cdot5^(-2))$