hello
the question given is to find the inverse of a function
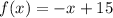
the inverse of the function is

step 1
replace every f(x) with y just like we've done above
step 2
replace every y with x and x with y in the equation
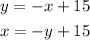
step 3
solve for y
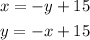
step 4
replace every y with f^-1(x)

the inverse of the function is f^-1(x) = -x + 15