To find:
Change in the frequency if the wavelength is reduced by a factor of 4.
Step-by-step explanation:
The speed of a wave is given by,
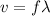
Where f is the frequency and λ is the wavelength of the wave.
If the wavelength is reduced by a factor of 4, then,
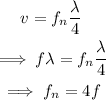
From above calculation, it is clear that the new frequency will be 4 times the old one.
Final answer:
Thus the correct answer is option D.